Description
1908: “I have made the following discovery and I pass it on to the world; The evolution of culture is synonymous with the removal of ornament from utilitarian objects.” – Loos
2022: “I have made the following discovery and I pass it on to the world; The removal of ornament from objects contradicts Nature and the Laws of Mathematics.” – Miles
Adolf Loos can be forgiven for being ignorant of the universal truth of ornamentation. He died before the mathematician Benoit Mandelbrot discovered the Mandelbrot set of points and fractals in 1979, well after Loos’ death in 1933.
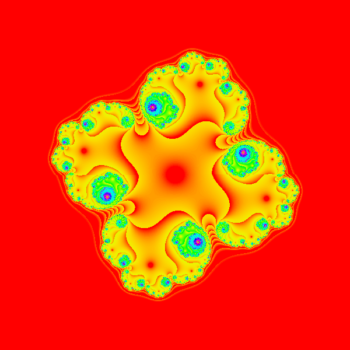
An ornament is a decoration used to embellish parts of a building or object. The Mandelbrot set is a mathematical object that clearly shows decoration[1]. The Mandelbrot set is generated through the application of a simple mathematical function. A graph of the set shows the boundary between the numbers inside the set and those outside. Decoration is a verified or indisputable calculation of the boundary of this set; it is a mathematical truth.
Descartes always considered mathematical demonstrations among the most evident truths that human mind can attain[2]. No matter how closely you zoom in and calculate the Mandelbrot set boundary you will always see more and more decorations. If a mathematical object shows infinite decoration then ornamentation cannot be criminal in use, but is rather a mathematical truth.
Furthermore, nature is full of fractals[3]. Flowers, ferns, leaves, river channels, lightning, and snowflakes are some examples of fractals in nature. If natural objects show fractal decorations then ornamentation cannot be criminal in use, but is rather a natural truth.
I present “The Mandelbrot Cube”, a utilitarian cubic form that illustrates through decorative fractal shapes the universal truth of ornamentation.
The 2 inch square Mandelbrot Cube was constructed from 18 gauge copper sheet. Each face was etched with the image of a fractal shape generated from my Fractal Image Explorer[4] computer program. The images were printed and transferred to the copper and etched with ferric chloride. The etched images were filled with a low temperature heat cured decorative enamel.
The cube is set on top of a 5 inch tall 3/8 inch diameter solid brass rod mounted in a white bronze cast footing. The finished Mandelbrot Cube sculpture is sealed with clear lacquer and attached to a 12x9x3 cm polished granite base.
Bibliography
[1] Robert L. Devaney (2006), Unveiling the Mandelbrot Set, in Plus Magazine.
[2] René Descartes (1628), Rules for the Direction of the Mind (Regulae ad Directionem Ingenii), in The Philosophical Writings of Descartes, Cambridge University Press 2012.
[3] Voss, R.F. (1988), Fractals in nature: From characterization to simulation, in The Science of Fractal Images.
[4] Miles, W. (2015), Fractal Image Explorer, Bronze Art by William Miles, https://www.bronzeart.ca/fractals/
[5] Miles, W. (2022), Ornament & Crime, Bronze Art by William Miles, https://www.bronzeart.ca/ornament-crime/